
If the core remnant has a mass greater than 3 solar masses, then not even the super-compressed degenerate neutrons can hold the core up against its own gravity. Gravity finally wins and compresses everything to a mathematical point at the center. The point mass is a black hole. Only the most massive, very rare stars (greater than about 25 solar masses) will form a black hole when they die. As the core implodes, it briefly makes a neutron star for just long enough to produce the supernova explosion.

Since the mass is in the top of the fraction, the escape velocity is greater for larger masses. The escape velocity is smaller for larger distances from the center because the distance is in the bottom of the fraction. The Earth's escape velocity at its surface is about 11 kilometers/second and the Sun's surface escape velocity is about 620 kilometers/second. White dwarfs and neutron stars have very large surface escape velocities because they have roughly the mass of the Sun packed into an incredibly small volume. A solar mass white dwarf has a radius of only 8800 kilometers, so its surface escape velocity is about 5500 kilometers/second. A solar mass neutron star would have a radius of just 17 kilometers, so its surface escape velocity would be an incredible 125,000 kilometers/second! (Real neutron stars have masses above 1.4 solar masses and smaller radii, so their escape velocities are even larger!)
A black hole probably has no surface, so astronomers use the distance at which the escape velocity equals the speed of light for the size of the black hole. This distance is called the event horizon because no messages of events (via electromagnetic radiation or anything else) happening within that distance of the point mass make it to the outside. The region within the event horizon is black from our viewpoint outside (if you were inside the event horizon, you could see all sorts of light). Rearranging the formula above for the escape velocity and putting in the speed of light c for the escape velocity, you find the event horizon is at a distance of
Black holes have been portrayed as cosmic vacuum cleaners in Hollywood films, sucking up everything around them. Black holes are dangerous only if something gets too close to them. Because all of their mass is compressed to a point, it is possible to get very close to them and still be outside all of the mass. Recall that gravity is an inverse square law force, so at very small distances, the strength of gravity around a point mass becomes very large. But objects far enough away will not sense anything unusual. If the Sun were replaced by a black hole of the same mass, the orbits of the planets would remain unchanged (it would be much colder in the solar system, though!).
Within the event horizon of a black hole, spacetime is so curved that any light emitted is bent back to the point mass. Karl Schwarzschild worked out the equations in General Relativity for a non-rotating black hole and found that the light rays within a certain distance of the point mass would be bent back to the point mass. The derived distance is the same as the event horizon value above. The event horizon is sometimes called the Schwarzschild radius in his honor.
Falling toward a black hole would not be a pleasant experience. If you fell in feet-first, your body would be scrunched sideways and stretched along the length of your body by the tidal forces of the black hole. Your body would look like a spaghetti noodle! The stretching happens because your feet would be pulled much more strongly than your head. The sideways scrunching happens because all points of your body would be pulled directly toward the center of the black hole. Therefore, your shoulders would be squeezed closer and closer together as you fell closer to the center of the black hole. The tidal stretching/squeezing of anything falling into a black hole is an effect conveniently forgotten by Hollywood movie writers and directors. [However, the tidal effect at the event horizon of a supermassive black hole (one with at least a million times the mass of the Sun) is much less than at the event horizon of a stellar-mass black hole, so an astronaut could still be alive crossing the event horizon of a supermassive black hole. At their respective event horizons, the tidal strength scales as (small black hole mass / big black hole mass)2.]
Your friend watching you from a safe distance far from the black hole as you fell in would see your clock run slower and slower as you approached the event horizon. This is the effect of "time dilation" (see General Relativity predictions section). In fact, your friend would see you take an infinite amount of time to cross the event horizon---time would appear to stand still. However, in your reference frame your clock would run forward normally and you would reach the center very soon. If you beamed back the progress of your journey into a black hole, your friend would have to tune to progressively longer wavelengths (lower frequencies) as you approached the event horizon. This is the effect of "gravitational redshift" (see General Relativity predictions section). Eventually, the photons would be stretched to infinitely long wavelengths.
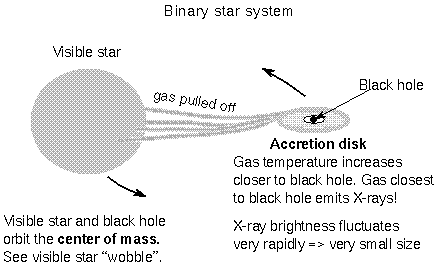
However, measuring the masses of all of the binary systems in the Galaxy would take much too long a time---there are over a hundred billion binary systems in the Galaxy! Even if it took you just one second to somehow measure a binary's total mass and subtract out one star's mass, it would take you over 3000 years to complete your survey. How could you quickly hone on the binary system that might have a black hole? Fortunately, black holes can advertise their presence loud and clear with X-rays.
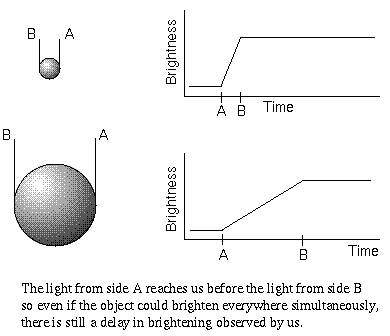
To make rapidly varying X-rays, the unseen companion must be small! The fluctuation timescale gives us the maximum possible diameter of the object. Since the speed of light is finite, it takes a given amount of time for light to travel across the object. The time it takes for any interaction to occur is diameter/speed, where the speed can be up to the speed of light if the object could somehow brighten everywhere simultaneously. The diameter = (time interval) × speed. The maximum diameter possible is for a speed equal to the speed of light. The quicker the fluctuations are, the smaller the object must be.
|
 |
Black holes themselves are invisible, but they produce very visible effects on nearby objects. Several stellar-mass black hole candidates have been found. Examples include Cygnus X-1 and V404 Cygni in the constellation of Cygnus, LMC X-3 in the constellation Dorado, V4641 Sgr in Sagittarius, J1655-40 in Scorpius, and the closest one, V616 Mon in the Monocerotis constellation is about 5100 light years away. Recently, astronomers have used the differences in how neutron stars and black holes accrete material from their accretion disks to show that black holes exist. Material falling toward a neutron star will emit x-rays as it spirals inward and also as it impacts onto the neutron star's surface. But material falling toward a black hole does not impact a surface; it vanishes through the event horizon. Comparing the brightness of compact objects heavier than 3 solar masses with those lighter than 3 solar masses, shows that the heavier masses are dimmer than the lighter masses even if they have the same orbital periods. Since the accretion rate should be the same for two objects with the same orbital period, the faintness of the heavier masses can only be explained if they are swallowing matter and energy like a black hole would do. In the Other Galaxies chapter more strong evidence is given for the existence of black holes in that "supermassive" black holes provide a simple explanation for the extremely energetic nuclei of peculiar galaxies called "active galaxies" .
Finally, the characteristics of the gravitational waves we detect from the merger of binary black holes or black hole and neutron star merger are what would be produced if the merging objects had such extreme densities. The gravitational waves would have a different shape if they were not produced by black holes. The black holes detected by the gravitational wave observatories are significantly larger than the black holes detected by X-ray observatories. It is possible that black hole mergers could explain the apparent gap between typical stellar-mass black holes and supermassive black holes residing at the centers of many galaxies.
![]() Go back to previous section --
Go back to previous section --
![]() Go to next section
Go to next section
last updated: October 31, 2024