Video lecture for this chapter
To measure distances on the imaginary celestial sphere, you use ``angles on the sky'' instead of meters or kilometers. There are 360 degrees in a full circle and 90 degrees in a right angle (two perpendicular lines intersecting each other make a right angle). Each degree is divided into 60 minutes of arc. A quarter viewed face-on from across the length of a football field is about 1 arc minute across. Each minute of arc is divided into 60 seconds of arc. The ball in the tip of a ballpoint pen viewed from across the length of a football field is about 1 arc second across.
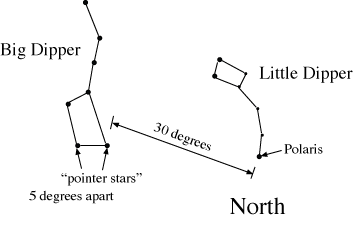
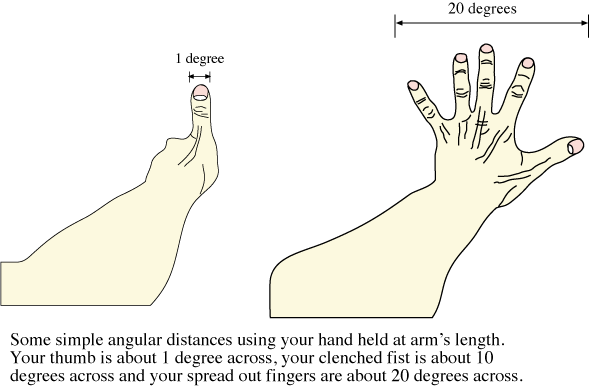
The Sun and Moon are both about 0.5 degrees = 30 arc minutes in diameter. The pointer stars in the bowl of the Big Dipper are about 5 degrees apart and the bowl of the Big Dipper is about 30 degrees from Polaris, the North Star that is very close to the North Celestial Pole. Some angles using your hand held at arm's length are described in the figure below. The arc from the north point on the horizon to the point directly overhead to the south point on the horizon is 180 degrees, so any object directly overhead is 90 degrees above the horizon and any object ``half-way up'' in the sky is about 45 degrees above the horizon.
Go back to previous section --
Go to next section
last updated: January 18, 2022