This material (including images) is copyrighted!.
See my copyright notice for fair use practices.
The area swept out by a line connecting an orbiting object and the central point
is the same for any two equal periods of times. That line is called a
radius vector in the following discussion.
The rate of
change of the swept-out area does NOT change with time. The line along which
gravity acts is parallel to the radius vector. This means that
there are no torques disturbing the
angular motion and, therefore, angular momentum is conserved. The part of the orbital
velocity (v-orbit) perpendicular (at a right angle) to the radius vector
(r) is vt. The rate of
change of the swept-out area = r×vt/2.
To calculate the orbital angular momentum use vt
for the velocity. So, the angular momentum = mass × vt
× r
= mass × 2 × (rate of change of area). That value does not change over time.
So if r decreases, v-orbit (and vt) must increase! If
r increases, v-orbit (and vt) must decrease. This is
just what Kepler observed for the planets!
 The total angular momentum = spin angular momentum + orbital angular momentum. The total
angular momentum is CONSTANT. To find the
spin angular momentum, subdivide the object into small pieces of mass and find the
angular momentum for each of the small pieces. Then add up the angular momentum
for all of the pieces. The Earth's spin speed
is decreasing so its spin angular momentum is DEcreasing. Therefore, the Moon's orbital
angular momentum must compensate by INcreasing. It does this by increasing the Earth-Moon
distance.
The total angular momentum = spin angular momentum + orbital angular momentum. The total
angular momentum is CONSTANT. To find the
spin angular momentum, subdivide the object into small pieces of mass and find the
angular momentum for each of the small pieces. Then add up the angular momentum
for all of the pieces. The Earth's spin speed
is decreasing so its spin angular momentum is DEcreasing. Therefore, the Moon's orbital
angular momentum must compensate by INcreasing. It does this by increasing the Earth-Moon
distance.

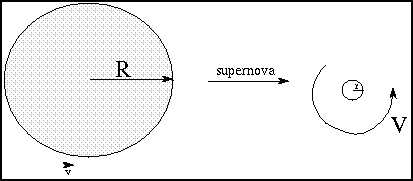
Originally, a big star has a core
10,000's to 100,000's kilometers in radius (the whole star is even bigger!).
Here the radius is used instead of the diameter, because what is
important is how far each piece of the core is from the spin axis that goes
through the exact center of the star.
The core spins at 2 to 10 kilometers/second at the core's equator. If no
external forces produce
torques, the angular momentum is constant. During a supernova the outer layers
are blown off and the core shrinks to only 10 kilometers in radius! The core angular
momentum is approximately = 0.4×M×V×R and the mass M
has stayed approximately the same. When the radius R
shrinks by factors of 10,000's, the spin speed
V must increase by 10,000's of times.
Sometimes the neutron star suddenly
shrinks slightly (by a millimeter or so) and it spins faster. The
neutron star produces radiation from its strong magnetic
field. This radiation is produced at the expense of the rotational energy and the
angular momentum is not strictly conserved---it slowly decreases. Therefore, the
neutron star spin speed slowly decreases.
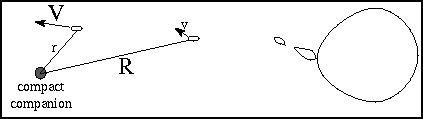
 Gas flowing from one star falls toward its compact companion into an orbit around
it. The orbital angular momentum is conserved, so as the gas' distance from the compact
companion DEcreases, its orbital speed must INcrease. It forms a rapidly rotating
disk-like whirlpool called an accretion disk. Over time some of the gas in the
disk gas give torques to other parts of the disk's orbital motions through friction. This
causes their angular momentum to decrease. Some of that gas, then, eventually falls onto
the compact companion.
Gas flowing from one star falls toward its compact companion into an orbit around
it. The orbital angular momentum is conserved, so as the gas' distance from the compact
companion DEcreases, its orbital speed must INcrease. It forms a rapidly rotating
disk-like whirlpool called an accretion disk. Over time some of the gas in the
disk gas give torques to other parts of the disk's orbital motions through friction. This
causes their angular momentum to decrease. Some of that gas, then, eventually falls onto
the compact companion.
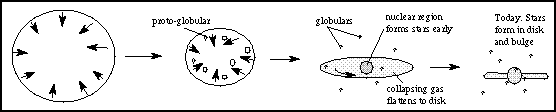
 A huge slowly spinning gas cloud collapses. Parts of
the roughly spherical gas cloud break up into small chunks to form stars and
globular clusters. As the rest of the gas cloud collapses, the inner,
denser parts collapse more rapidly than the less dense parts. Stars form
in the inner, denser parts before they form in the outer, less dense parts.
A huge slowly spinning gas cloud collapses. Parts of
the roughly spherical gas cloud break up into small chunks to form stars and
globular clusters. As the rest of the gas cloud collapses, the inner,
denser parts collapse more rapidly than the less dense parts. Stars form
in the inner, denser parts before they form in the outer, less dense parts.
All the time as
the cloud collapses, the spin speed must increase. Since no outside
forces produce torques, the angular momentum is conserved. The rapidly spinning
part of gas cloud eventually forms a disk. This is because the cloud can
collapse more easily in a direction parallel to the spin axis. The gas that
is orbiting perpendicular to the spin axis has enough inertia to
resist the inward pull of gravity (the gas feels a ``centrifugal force''). The
densest parts of the disk will form stars.
 Go back to previous section
Go back to previous section
last updated: 28 May 2001
Is this page a copy of Strobel's
Astronomy Notes?
Author of original content:
Nick Strobel





