Video lecture for this chapter
Early astronomy concentrated on finding accurate positions of the stars and planets. This was due in part to the influence of astrology, but later, accurate positions came to be important for determining the physical characteristics of the stars and planets. Accurate positions for the stars was also crucial for commercial and military navigation (navigation by the stars has only recently been replaced by the use of satellite systems such as the Global Positioning System). But probably of more importance to you is where to point your telescope or binoculars to find that cool object talked about in the newspaper or astronomy magazine.
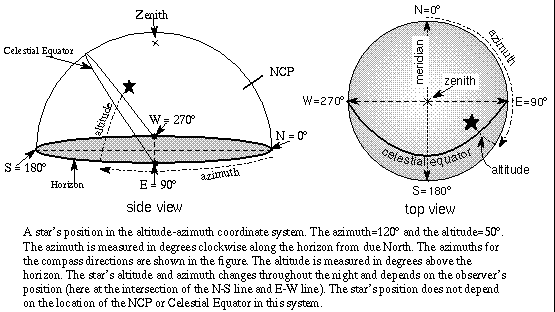
There are a couple of popular ways of specifying the location of a celestial object. The first is what you would probably use to point out a star to your friend: the altitude-azimuth system. The altitude of a star is how many degrees above the horizon it is (anywhere from 0 to 90 degrees). The azimuth of a star is how many degrees along the horizon it is and corresponds to the compass direction.

Azimuth starts from exactly North = 0 degrees azimuth and increases clockwise: exactly East = 90 degrees, exactly South = 180 degrees, exactly West = 270 degrees, and exactly North = 360 degrees = 0 degrees. For example, a star in the southwest could have an azimuth between 180 degrees and 270 degrees. Since stars change their position with respect to your horizon throughout the night, their altitude-azimuth position changes. Also, observers at different locations looking at the same star at the same time will see it at a different altitude-azimuth position. A concise summary of this coordinate system and the numbers involved is given at the end of this section.
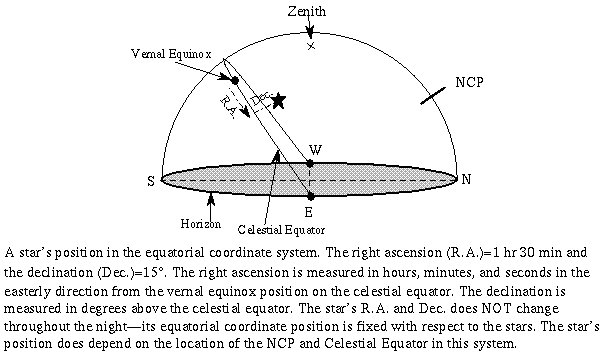
The second way of specifying star positions is the equatorial coordinate system. This system is very similar to the longitude-latitude system used to specify positions on the Earth's surface. This system is fixed with respect to the stars so, unlike the altitude-azimuth system, a star's position does not depend on the observer's location or time. Because of this, astronomers prefer using this system. You will find this system used in astronomy magazines and in most sky simulation computer software.
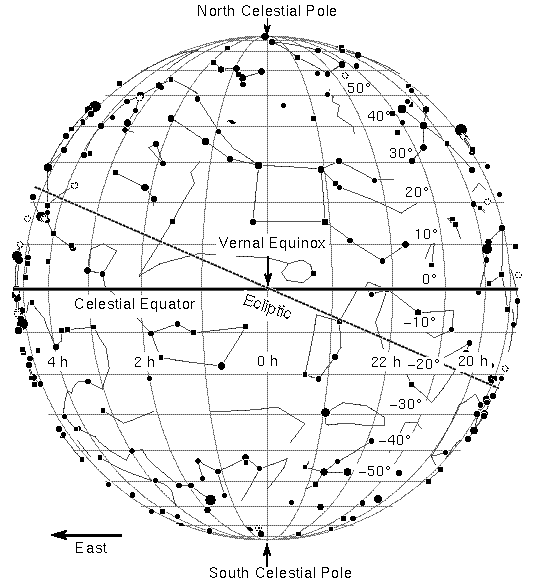
The lines on a map of the Earth that run north-south are lines of longitude and when projected onto the sky, they become lines of right ascension. Because the stars were used to measure time, right ascension (RA) is measured in terms of hours, minutes, and seconds instead of degrees and increases in an easterly direction. For two stars one hour of RA apart, you will see one star cross your meridian one hour of time before the other. If the stars are not circumpolar, you will see one star rise one hour before the other. If they were 30 minutes of RA apart, you would see one rise half an hour before the other and cross your meridian half an hour before the other. Zero RA is where the Sun crosses the celestial equator at the vernal equinox. The full 360 degrees of the Earth's rotation is broken up into 24 hours, so one hour of RA = 15 degrees of rotation. The lines of RA all converge at the celestial poles so two stars one hour of RA apart will not necessarily be 15 degrees in angular separation on the sky (only if they are on the celestial equator will they be 15 degrees apart).
The lines on a map of the Earth that run east-west parallel to the equator are lines of latitude and when projected onto the sky, they become lines of declination. Like the latitude lines on Earth, declination (dec) is measured in degrees away from the celestial equator, positive degrees for objects north of the celestial equator and negative degrees for objects south of the celestial equator. Objects on the celestial equator are at 0 degrees dec, objects half-way to the NCP are +45 degrees, objects at the NCP are +90 degrees, and objects at the SCP are -90 degrees. Polaris's position is at RA 2hr 31min and dec 89 degrees 15 arc minutes. A concise summary of this coordinate system and the numbers involved is given at the end of this section.
The Basic Coordinates module of the University of Nebraska-Lincoln's Astronomy Education program provides a great way to make the connection between terrestrial coordinates (longitude and latitude) and the equatorial coordinate system (link will appear in a new window). The first part of the module has you drag a cursor around on a flat world map or globe and read off its terrestrial coordinate position. The second part of the module has you do the same sort of thing using a flat map of the sky or a globe of the celestial sphere and read off the right ascension and declination. Both parts also illustrate the distortion that happens when you project a curved spherical surface onto a flat two-dimensional map.
The UNL Astronomy Education's Rotating Sky module has you explore the connection between the two coordinate systems. You can change your location on the Earth and adjust the position of multiple stars and see where the stars would appear and how they would move on the celestial sphere and around your position on the Earth as the Earth rotates beneath the stars.
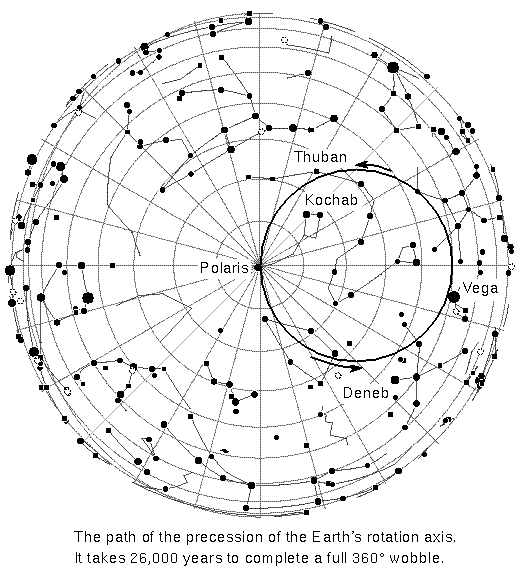
An effect called precession causes the Sun's vernal equinox point to slowly shift westward over time, so a star's RA and dec will slowly change by about 1.4 degrees every century (a fact ignored by astrologers), or about 1 minute increase in a star's RA every twenty years. This is caused by the gravitational pulls of the Sun and Moon on the Earth's equatorial bulge (from the Earth's rapid rotation) in an effort to reduce the tilt of the Earth's axis with respect to the ecliptic and the plane of the Moon's orbit around the Earth (that is itself slightly tipped with respect to the ecliptic). Like the slow wobble of a rapidly-spinning top, the Earth responds to the gravitational tugs of the Sun and Moon by slowly wobbling its rotation axis with a period of 26,000 years.
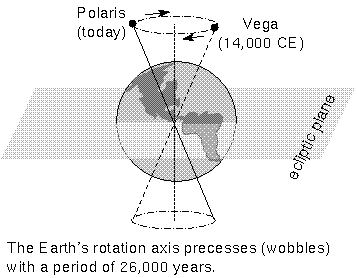
This motion was first recorded by Hipparchus in 100 B.C.E. who noticed differences between ancient Babylonian observations and his own. When the Babylonians were the world power in 2000 B.C.E., the vernal equinox was in the constellation Aries and the star Thuban (in Draco) was the closest bright star to the NCP. At the time of Jesus Christ the vernal equinox had shifted to the constellation Pisces and the star Kochab (in the bowl of the Little Dipper) was the closest bright star to the NCP. Now the star Polaris is close to the NCP and the vernal equinox is close to the border between Pisces and Aquarius (in 2600 C.E. it will officially be in Aquarius) which is what a popular song of years ago refers to with the line ``this is the dawning of the Age of Aquarius''. In the year 10,000 C.E., the bright star in the tail of Cygnus, Deneb, will be the pole star and Vega (in Lyra) will get its turn by the year 14,000 C.E. Many horoscopes today are still based on the 4,000-year old Babylonian system, so even though the Sun is in Aries on my birthday, the zodiac sign used for my horoscope is Taurus. I guess it's hard to keep up with all of the changes in the modern world! (However, remember from the discussion about astrology in the first chapter, that the problem with astrology is not whether the astrologers take into account precession or not but, instead, the problem with astrology is when astrology is claimed to be a science.)
| altitude | azimuth | declination |
|---|---|---|
| precession | right ascension |
![]() Go back to previous section --
Go back to previous section --
![]() Go to next section
Go to next section
last updated: January 18, 2022